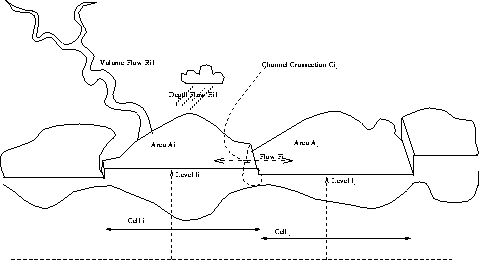
Figure 2: Schematic view of the cell based lake salinity model showing some of the major parameters.
The use of, setup and running the lake model is described in the accompanying user manual. The user manual is available in paper form and on the world wide web.
The St. Lucia lake salinity model is essentially a model of flows of water and salt from thoroughly mixed cells to thoroughly mixed cells governed by linear level dependent gravity flows.
Figure 2: Schematic view of the cell based lake salinity model showing
some of the major parameters.
The flow from cell i to cell j is given by 
. (See figure 2).
Where 
is the level in cell i, and 
is the cross
sectional area of the channel between i and j and 
is the relaxation
time constant. R is a fitted parameter, related to a roughness coefficient. This is under
the ideal condition of a lake system. However, for the modeling of the Douglas Weir
system, the behaviour in some cells ceased to be that of a lake (lake level above the
bottom of both cells), and became more like a river. ie. Water level in one cell below the
cell bottom of the adjacent cell. In that case the model has the rule that, if the bottom
of cell i is greater than the level of cell j, then the flow is proportional to the level
in cell i minus the bottom of cell i.
The rate of change of volume in cell i is given by :-
Where 
is the set of all neighbouring cells of cell i, and 
is the set of all
volume in/out flows such as rivers and estuaries. 
is the set of all
depth flows such as rainfall and evaporation. 
is the area of cell
i at the current level . 
is the volume of flow from source k to cell i at time t, given
that the level in cell i is . 
is the depth of flow from source m to cell i.
The salt load transported from cell i to cell j is 
:=
Where 
is the salt concentration in cell i if the flow is from cell i to j, else it
is the salt concentration in cell j if the flow is from cell j to cell i.
